Extreme Diabolic Tough of April 12, 2007 Page 1

The following illustrated proof for the diabolical & extreme Tough Sudoku of April 12, 2007 employs a wide array of techniques. Of special import for this puzzle are Advanced Forbidding Chains . By this I mean that a technique, including possibly a Forbidding Chain (also called Alternating Inference Chain (AIC) is used as an argument in the chain. If this is your first visit to this blog, you are strongly encouraged to research how I use Forbidding Chains, as this puzzle is a slight bit hard.

Previous blog pages may be helpful. Links to these pages are found to the right, under Sudoku Techniques. The list is growing, so specifically, one may want to refer to the following previous blog pages:

• Locked Candidates
• Pairs, Triples, Quads
• Coloring
• Finding Coloring
• Y Wing Styles
• Finding Y Wing Styles
• Forbidding Chains 101 The Theory
• Forbidding Chains 102 The Practice How to Find 'em
• Advanced Forbidding Chains The theory of using techniques within Chains
• Definitions: Most terms used on this page have a defintion somewhere on the defintions page.

The illustrations of forbidding chains, also called Alternating Inference Chains (AIC), shown in this proof will share this key:

• black line = strong inference performed upon a set (strong link)
• red line = weak inference performed upon a set (weak link)
• black containers define a partioning of a strong set(s)
• candidates crossed out in red = candidates proven false

Please be aware that, for me, strong and weak need not be mutually exclusive properties.

The Puzzle

Hidden Pair 78

In row 6, only two places remain for both candidate 7 and candidate 8. Thus, if any other candidate were to exist there, too few places would remain for at least one of candidate 7,8. I have illustrated the elimination as a wrap around, or continuous nice loop forbidding chain:

• b6=7 == h6=7 -- h6=8 == b6=8 => bh6=78.

Locked candidate 9

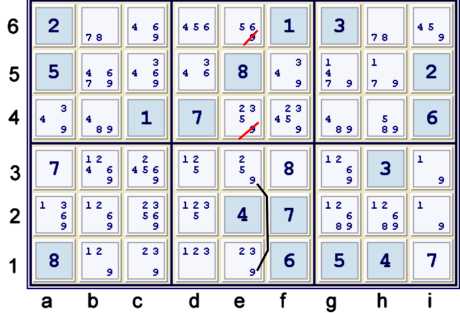
Since the 9s in box e2 exist only within column e, if a 9 were to exist in column e outside of box e2, there could be no 9s in box e2. Thus, one can eliminate the possible 9s shown above. I have also illustrated this elimination as a very short forbidding chain:

• e1=9 == e3=9 => e46≠9

Naked Pair 19

Since cells i23 are both limited to only candidates 1 and 9, all the cells which see both i23 cannot contain 1 or 9. The logic is almost exactly the same as for the hidden pair explained earlier. The wrap around forbidding chain is also very similar, but there are subtle differences. A very good understanding of how forbidding chains work can be achieved by studying such simple techniques. As a forbidding chain:

• i2=1 == i2=9 -- i3=9 == i3=1 => gh2,h3,i678 ≠ 19

After making these eliminations, one can solve another cell: c6 = 9% row.

Coloring with candidate 3

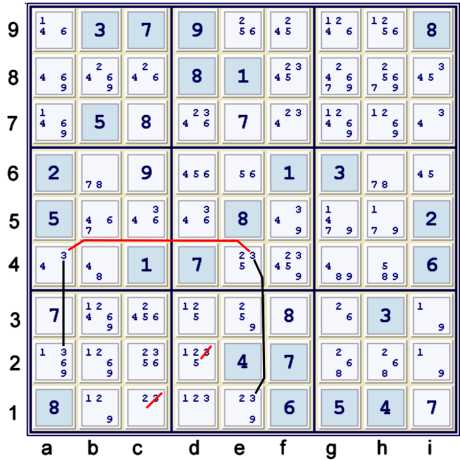
I call any technique which considers exactly one type of candidate coloring. Coloring is also a great place to start learning about forbidding chains. Above, the 3's in columns a and e are sufficient information to justify the eliminations shown. As a forbidding chain on only candidate 3:

• a2 == a4 -- e4 == e1 => c1,d2≠3

A simple forbidding chain like this one reduces to: a2=3 == e1=3, which means that at least one of a2,e1 must be 3. Thus, all the possible 3s which see both of the cells a2,e1 are eliminated.

Two more cells are now solved: c1 = 2% cell and then b8 = 2% row, column & box.

Typical forbidding chain using candidates 345

Most easy techniques have been exhausted. To advance the puzzle a bit further, I used the chain illustrated above:

• c8=4 == a789=4 -- a4=4 == a4=3 -- a2=3 == c2=3 -- c2=5 == c3=5 => c3≠4

The first part of the chain, c8=4 == a789=4 is a type of grouped argument that is frequently helpful. One may note that a789=4 == a4=4 would also be a true statement. The fact that the relationship between a789=4 and a4=4 is both a strong inference and a weak inference is of little concern. This is also the case with a4=3 & a2=3. Please review the blog pages on forbidding chain theory if this causes confusion.

After making the elimination of 4 from c3, the following Unique Possibilities are available:

• b3 = 4% box & row
• b4 = 8% cell
• b6 = 7% cell
• b5 = 6% cell
• h6 = 8% cell
• g2 = 8% row & box

Pair 34

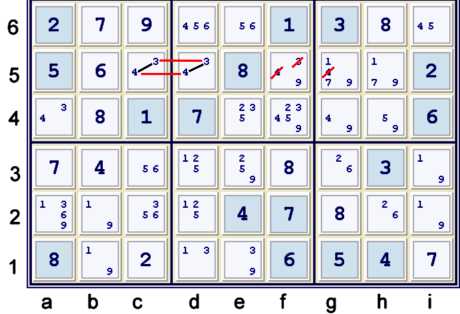
Illustrated above is the newly uncovered naked pair 34 at cd5. After making the indicated eliminations, f5=9% cell.

Very Common Y Wing Style

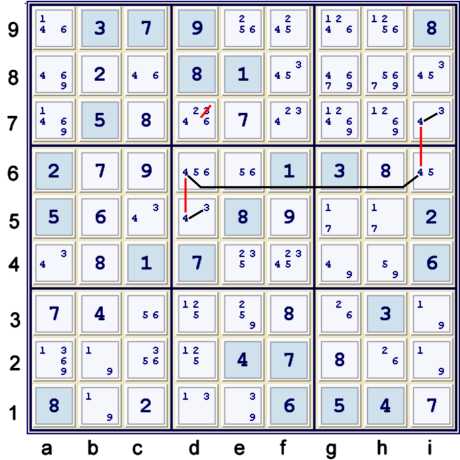
The cells d5 = i7 = 34 are common markers for one to look for this elimination. The 4s in row 6 serve as the vertex, or bridge, between these two cells, allowing the elimination indicated above. As a forbidding chain, this very common and very easy to find technique looks like:

• d5=3 == d5=4 -- d6=4 == i6=4 -- i7=4 == i7=3 => d7≠3

After making this elimination, the 3s in box e8 exist only in column f, thus one can additionally say:

• f7=3 == f8=3 => f4≠3
• or, alternatively: Locked 3s at f78 => f4≠3

Naked Pair 19

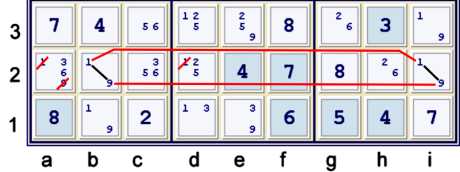
The naked Pair 19 at bi2 justifies the eliminations shown above.

This concludes the first page of this puzzle proof. Some possible headaches, and certainly some very interesting stuff, can be found on the next page.