The following very sparselyillustrated solution for the potentially instructive
Tough Sudoku of August 8, 2009 is intended only to further explore the concept
of atypical groupings as Quantum sets. This particular advanced Sudoku
solving strategy, tip or trick can be relatively simple to employ and to visualize.
If this is your first visit to this blog, welcome! Unfortunately, if you are a first time visitor, this page may seem
like it is written in a different language. Well, it is!! Previous blog pages may be helpful. Links to these pages are
found to the right, under Sudoku Techniques. The earliest posts are at the bottom, and if you have never perused
the intricacies of our special coded language here, you may wish to start close to beginning. The list is rather large, so below
find a list of links that may be pertinent to this particular puzzle.
Since a fully illustrated solution often takes quite a bit of time and work, and since I am fundamentally lazy, today's solution will only be
sparsely illustrated. The main point of this post is to highlight just one advanced concept: Quantums. The term typically refers to SIS created
by a Uniqueness pattern. However, it is expanded herein to include any atypical SIS group. This is a rather broad definition, and as such may be often of
little practical use. Nevertheless, there are some relatively simple - and relatively common - groupings that can be broadly exploited. Of course, one does
often find what one seeks....
The Puzzle
For those whom do wish to view a full solution path, I have posted at the bottom of this page a group of steps sufficient to slay this puzzle.
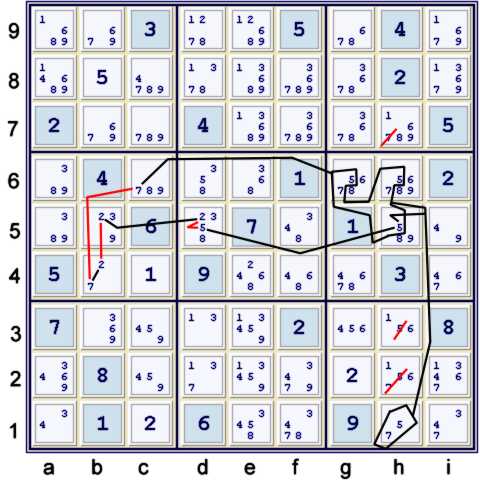
Three UPs are available here. At this point, the Quantum deduction is available. However, the next picture includes a puzzle state where the
following steps have been taken:
- Start 23, UP 26
- (5Skyscraper): d5 = h5 - h1 = e1 => d23,e6 ≠5
- (5)g3 = (5)g6 - (5)h5 = (5-2)d5 = (2-3)b5 = (3)b3 => g3≠3, b3≠5
- The step above is significant in one additional manner: As an AIC, it is immediately clear that one achieves both eliminations listed.
A proof by contradiction approach would often fail to get both these eliminations.
- UP 27
An Almost Quantum Naked Pair used in a chain
Illustrated (poorly, perhaps) below is an example of thinking in groups. This is primarily, to me at least,
what Quantums entail. One could equivalently view the deduction below as an Almost Y Wing Style loop. Also, one could describe it
as using an Almost Hub Spoke and Rim pattern. Awareness of these alternative viewpoints may be helpful. However, one can distill
the logic contained in all of these more simply.
- Candidate (5) in box h5 is limited to Column h and cell g6.
- Candidate (7) in row 6 is limited to Column h, cell g6, and cell c6
- There exists an ALS (5=7) in Column h - in this case at the cell (57)h1
- Cell g6 can ultimately contain no more than one of (57)
- Considering candidate (7) in row 6, candidate (5) in box h5, and (57)h1,
- It is clear that we have [Quantum Naked Pair (57)h156.g6 = (7)c6]
I include the
.g6 because (5-7)g6 is crucial to the deduction. It should be fairly easy to
see that at least one of (57) at h56 is true, or (7) exists at c6. Thus, some sort of (NP57) exists at h156 OR (7)c6, or perhaps both.
This does not quite finish the deduction.
The entire chain can be written from either direction, so it is a true AIC, and not really a large
stretch in group thought. Here is the chain written in the less intuitive direction:
- (NP57)h15 = (5-2)d5 = (2)b5 - (2=7)b4 - (7)c6 = (QNP57)h156.g6
- => h2≠57, h3≠5, h7≠7
The chain works equally well considering candidate (7) in box h5 instead of candidate (7) in row 6.
Such an alternative presentation is common. Here is the alternative presenation, also reversed in
order, although this order reversal is arbitrary.
- (QNP57)h156.g6 = (7)gi4 - (7=2)b4 - (2)b5 = (2-5)d5 = (NP57)h15
Of course, the same eliminations are proved. This simple deduction happily serves to significantly
simplify the rest of the puzzle solution.
One can alternatively view this as a Kraken type step, considering all the possible locations for
candidate (7) in row 6. This viewpoint is almost identical in logic. However, In My Opinion, it tends to miss
the point of the simplicity involved in thinking in atypical types of groups.
Almost Unique Rectangles (AURs) create similar types of atypical groups. The next step that I
have chosen to discuss can be achieved by using either one, or both, of two AUR's.
SIS created by AURs, two examples from one position, both leading to the same conclusion.
Three steps were taken from the previous postion to get to the one shown below. Two AURs bear
consideration:
- (AUR16)ai89: Note that candidate (1) is already limited to ai89, therefor we have Almost
Hidden Pair (16)i89 and Almost Hidden Pair(16)a89. To avoid the Unique Rectangle,
- (AUR34)ai12: Note that we have almost Naked Pair (34) at both a12, i12. To avoid the Unique Rectangle,
Ultimately, to avoid either of these AUR's, we will end up avoiding both. This is a nice curiosity.
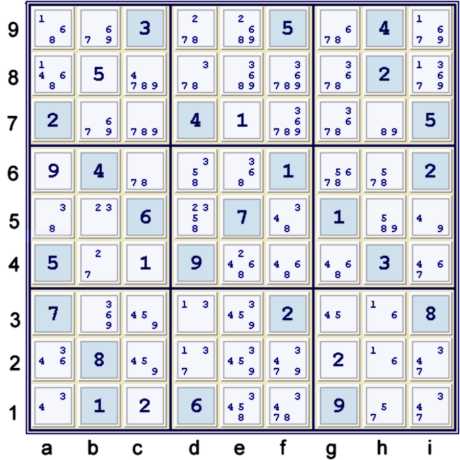
Two chains which reach the same conclusion are easy to spot:
- (6)a2 = (6-7)i4 = (7-2)b4 = (2-3)b5 = (3)b3 => b3≠6
- (6)a2 = (7)i12 - (7)i4 = (7-2)b4 = (2-3)b5 = (3)b3 => b3≠6
Interestingly enough, if (6) does not occur at a2, then both Unique Rectangles would be, in a sense, inevitable.
Naturally, since the puzzle has one solution, both Unique Rectangles will lead to some contradiction somewhere.
A Possible Solution Path using the Quantums listed above
Of course one can solve this puzzle without using the steps indicated above. Below is one way
to solve it using them:
- 1) Start 23 UP 26
- 2a) (Sky5): d5 = h5 - h1 = e1 => d23,e6≠5
- 2b) (5)g3 = (5)g6 - (5)h5 = (5-2)d5 = (2-3)b5 = (3)b3 => g3≠3, b3≠5, UP 27
- 3a) (NT459)a1.c23 = (NT389)a1.56 => a2,c6≠9 A very nice ALS x ALS with restricted common(3)
- 3b) (QNP57)h156.g6 = (7)c6 - (7=2)b4 - (2)b5 = (2-5)d5 = (NP57)h15 => h237≠57
- 3c) (NP16)h23 => g3,i2,h67≠16 UP 28
- 4) (7)h6 = (7-5)h1 = (5-4)g3 = (4)g4 pause - (4=9)i5 => h6≠9, g4≠7, UP 29
- 5) Note (AUR16)ai89 => (6)a2 = (6-7)i4 = (NT769)b489 => b3≠6, UP 33
- 6) (3)b3 = (3)e3 - (3)e6 = (3-5)d6 = (5-2)d5 = (2)b5 => b5≠3, UP 43
- 7) (NP57)h16 => h5≠5, UP81
SIS Specrtrum:
- Total SIS: 36
- Max Depth: 7 at step 5
- non-ssts steps: 6
- Rating: 2.85 Yikes!